THE ACOUSTICAL EFFECTS OF THE CORE PRINCIPLES OF THE BEL CANTO

METHOD ON CHORAL SINGING

Presented by Laurier Fagnan at Ircam, Paris, June 19th, 2002

Introduction

For several years now I have observed the positive vocal and acoustical effects that the vocal principles of the bel canto method can have on choral singing. These principles have been accepted and advanced for centuries in the field of opera and classical solo singing, but it is usually with much hesitation that choir directors consider applying these principles to the vocal technique of their ensembles. However, the study we have undertaken at Ircam (Institute of Research in Musical Acoustics) in Paris, has shed much light on this process and provided objective quantification of the possibilities offered by the effective application of the bel canto principles within the choral setting.

It has not been the aim of this study to compare choral singing technique with solo singing technique, nor to have choristers sing as though they were soloists. The goal has rather been to have choirs implement the time-proven principles of the bel canto method while respecting the norms of choral practice, i.e. blend, group intonation, etc., and to subsequently test the acoustic impact that these principles exercise on choral singing.

Although not a complete paper per se, this introductory document briefly outlines four of the eight principles which will be dealt with in greater detail in a doctoral thesis of the same title, and presents results through a series of acoustical analyses which offer objective acoustic quantifications of the application of bel canto principles within a choral environment.

All of the choral experiments in this study, with the exception of one, took place in the Espace de Projection at l'Ircam in Paris. A variety of choral ensembles was chosen, including a men's choir, a youth choir, a university mixed choir and a community mixed choir. The ensemble's own music director initially led the ensemble in the singing and recording of a series of approximately twelve exercises chosen for the study. It was made clear that these could be repeated as many times as possible and should be sung to the choir's best abilities. After this initial period, the choir worked on learning the bel canto principles and applying them to their singing. The same exercises were re-recorded during this process. Finally, the subsequent acoustical comparison of these two "before and after" versions of the same exercises led to the analyses below. In these analyses, the first window represents an exercise sung by a choir prior to having worked the principle in question, and the second window, the same exercise after having worked the bel canto principle at hand.

The programs used for these analyses, namely xspect, additive, and XSedit, which were developed at Ircam, as well as the assistance of Xavier Rodet, were extremely useful in helping me show, in an objective and scientific manner, the acoustical advantages of this method.

I. Vibration

The first analyses demonstrate how the coup de glotte proposed by Manuel Garcia can have a positive effect on the regularity of vibration and the fundamental frequency in choral singing (fig.1.1). This principle has unfortunately been one of the most widely misunderstood of all the bel canto vocal tenets, in large part because of the use of the term coup (stroke, hit). All that was originally meant by this term was that the initial vibration of the vocal folds was to begin from a closed, not an open, position. With this principle, choristers are encouraged to lightly close the glottis at the end of inspiration, just prior to the attack which begins in a controlled fashion from this closed position. As phonation continues, they are to maintain a feeling of consistent and collected vibration, as opposed to allowing the vibrating vocal folds to be spread apart by superfluous breath flow.

fig. 1.1 A choir singing [i], [a], [i], [a] to a3 (220Hz). 'x' axis=time, 'y'=frequency in Hertz.

After having worked the coup de glotte (bottom window, fig. 1.1), the choir is much more successful at beginning the attack in the centre of the pitch and capable of sustaining the fundamental frequency in a more stable and consistent manner.

Through implementation of the coup de glotte, regular vibration is also shown to have positive effects on a choir's spectral energy (fig 1.2):

fig. 1.2 Male chorus singing [i] . The spectrum in red represents the analysis after the application of the coup de glotte, black is before. The horizontal axis represents the frequency of the partials displayed while the vertical axis represents amplitude in decibels.

Of interest in this analysis (fig.1.2) is the fact that the first two partials are equal in amplitude, hence the choir sang at the same volume the first time. On the other hand, starting from this point, and especially in formant regions, there is a remarkable increase in the spectral energy of the more vibrant version. It is in the area of the singer's formant (2.3-3.5kHz), a spectral band in which the ear is the most sensitive, that the difference between the two examples is most remarkable. This shows the increased acoustic efficiency available to a choir which applies the regular, collected vibration espoused by the bel canto masters.

The spectrogram presents another way in which we can effectively show this difference (fig.1.3).

fig.1.3 Same choir and sound excerpt as in fig.1.2, with vocal difference displayed using a spectrogram. 'x' axis=time, 'y'=frequency.

This analysis similarly shows that there is as much if not more intensity in the area of the fundamental frequency in the first version (dark orange) yet much more energy in the higher frequencies in the second (bottom window). One can conclude that if a choir applies bel canto principles of vibration, it will not need to sing as "loudly" to reap the benefits of increased vocal intensity. It is also recognized that the presence of harmonic activity in the region of the singer's formant can have a very positive effect on vocal timbre as is demonstrated in the following sound example.

*** SOUND EXAMPLE (before) *** (adolescent chorus of men, women in background, singing [i], a3; these examples are for
*** SOUND EXAMPLE (after)*** figures 1.2-1.4)

If we take the same sound segment and isolate and measure only the energy in the frequency band between 2.3 and 3.5kHz , we very clearly see the spectral superiority of the more vibrant bel canto version (red dots, fig.1.4). With this analysis we can observe an overall 11dB gain in energy in the region of the singer's formant after the principle of regular vibration has been applied. Considering once again that the first partials of this sound segment were even in amplitude (fig. 1.2), one can conclude that this second attempt is acoustically much more effective.

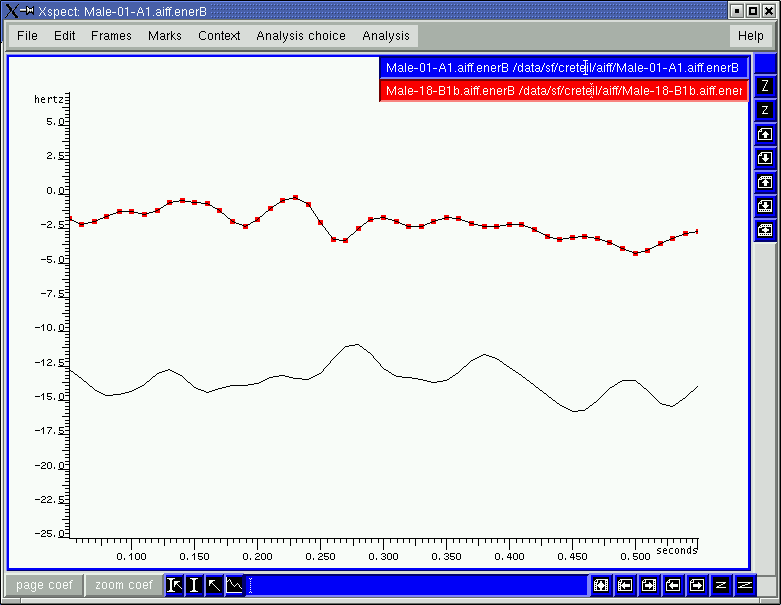
fig.1.4 Measurement of energy in the spectral band of 2.3 to 3.5kHz (singer's formant region). N.B. The 'y' axis should not read "hertz" but rather "dB" (intensity) , the 'x' axis is time. An 11dB gain in the 'after' version in red.

Much has been written concerning the importance of harmonic energy in the singer's formant region. There is also much documentation on the lack of energy in this range in choral singing. The ability to effectively extract and measure the spectral energy in this band using the EnerBande program was very useful to this study. It enabled us to objectively measure the energy in this region both before and after bel canto principles were implemented and assists in critically assessing much of what has been written on the vocal technique of choirs and, in particular, on how proper vocal vibration can help to provide adequate energy in this band. This will be of special interest to choirs which sing with orchestra as it has been shown that orchestral sound is not as strong in this region and that voices possessing strength in these upper frequencies can more easily be heard and discerned over an orchestra's sound.

II. Chiaroscuro Resonance ("clear-obscure" or "bright-warm")

It is a very well-known acoustical fact that all vowels were not created equal. Individual vowels do not resonate in quite the same way, or at same frequency. To effect a vowel change, the articulators must change position and the vocal tract alter its shape. These movements bring with them a multitude of muscular adjustments which often have a pejorative effect on the stability of the fundamental frequency. This phenomenon has often been observed and commented upon by vocal acousticians under the headings of "intrinsic pitch of vowels" and "articulatory F0 disturbances." For example, several experiments have shown that the change from [i] (heed) to [E] (head), is often accompanied by a rather significant drop in fundamental frequency. There are various theories which attempt to explain this correlation, one of them being the "tongue-pull" hypothesis which suggests that the higher tongue position required for frontal vowels might also cause a longitudinal stretching of the vocalis muscles which would tend to raise the fundamental frequency. ¹ Whatever the precise reason(s) might be, the relationship between changes in resonance and alterations in vibration of the vocal cords (frequency) seems to be quite strong.
Our research indicates, however, that the pejorative influence that necessary articulatory changes exercise on vocal vibration can be reduced, and in some cases removed, when the bel canto principle of chiaroscuro resonance is observed. Though certain vowels are incontestably more chiaro (bright) or oscuro (dark) than others by nature, this principle seeks to establish an equal proportion of brightness and darkness (or richness) across the complete vowel spectrum. Concentrating on equalizing the consistency of this ubiquitous "bright-dark" quality seems to even out the upper harmonic activity among the different vowels. It also helps to remove many of the extraneous and unnecessary articulatory changes made when changing vowels, thereby providing a more stable environment for the vibrating vocal folds. These elements of chiaroscuro resonance tend to play an important role in reversing the pejorative effect that articulatory changes can have on fundamental frequency, as demonstrated by the following analyses.

fig.2.1 Choir singing the vowels [i]-[E] (b e d)-[y] (French 'tu' )-[a] at approximately 1.5 second intervals along the 'x' axis, before chiaroscuro. Compare with fig. 2.2.

*** SOUND EXAMPLE (before) *** (fig.2.1)
*** SOUND EXAMPLE (after)*** (fig.2.2)

The red line of the fig. 2.1 and 2.2 is placed at the average frequency of the first vowel [i] as calculated by the Additive program developped at Ircam. We can easily note a significant drop in fundamental frequency of almost 2Hz coinciding with the lower jaw's descent while passing from [i] to the second vowel of [E] (at 1.5s, fig.2.1). The fundamental frequency rises when the jaw is closed again slightly for the [y] (at 3.2s.), and drops again for the last vowel change to [a] (at 5.5s.). This vocal-acoustical phenomenon corresponds very closely to the results of experiments carried out by Sten Ternstrom and Johan Sundberg in Stockholm. It is in fact the subject of their articles "Intonation Precision of Choir Singers," and "Articulatory f0 Perturbations and Auditory Feedback."¹

fig.2.2 Same choir as in fig.2.1 singing the vowels [i]-[E]-[y]-[a] after having worked the principle of chiaroscuro resonance balancing.
Compare with fig.2.1.

In the exercise leading to figure 2.2, choristers were asked to maintain the same amount of "brightness" in all of the four vowels. They experimented with the ability to maintain the upper partials in the sound while allowing the jaw to make the necessary movements for the different vowel sounds. Their attention was in no way drawn to the fact that there was an intonation problem, but rather a resonance problem. After having worked on the principle of chiaroscuro resonance for approximately fifteen minutes, it is evident that the articulatory perturbations which were exercising a negative influence on fundamental frequency stability in fig. 2.1 are all but gone in 2.2. The drop in pitch from the first to the last vowel was of 3.22Hz in figure 2.1 and is reduced to 0.29Hz in figure 2.2.

The following 'before' and 'after' examples exhibit the same positive effect with other choirs on a variety of exercises.

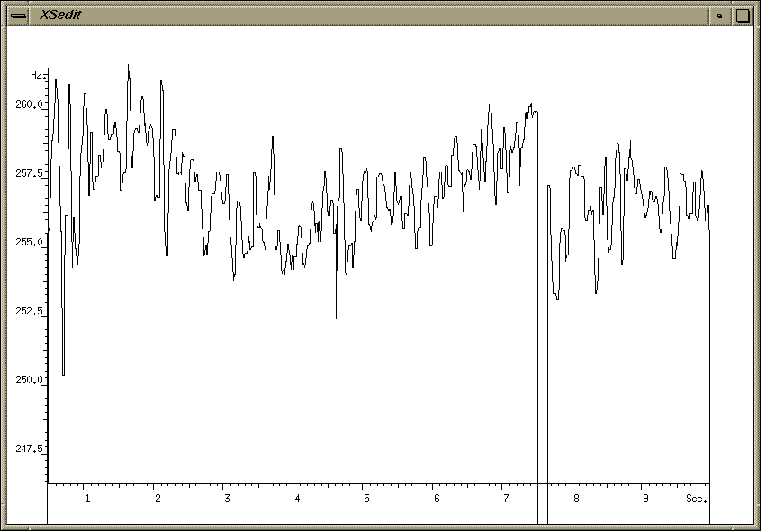
fig.2.3 Male choir singing the phrase Kyrie e leison before chiaroscuro, on middle C .

In figure 2.3, we clearly see the drop in pitch when moving from [i] to [E] at approximately 2.5s. Pitch rises as the chorus returns to [i] at 6s. before dropping once again for the [o] at 7.7s. Although these fluctuations are not completely eliminated in the 'after' version of figure 2.4, they are remarkably reduced.

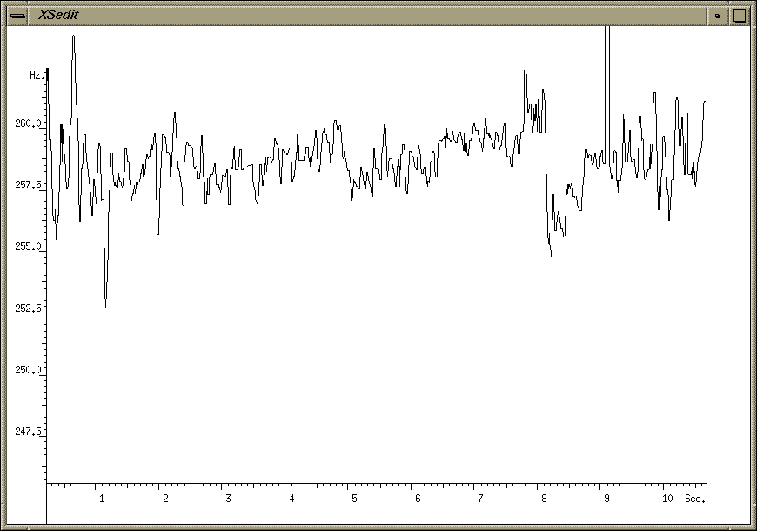
fig.2.4 Kyrie e leison after having worked chiaroscuro (same choir as in fig. 2.3).

fig.2.5 Adolescent male choir singing .Kyrie e leison 'before.'

We must however note that with the application of the chiaroscuro principle, there is sometimes a tendency to overcompensate for the tendency natural to drop for the darker vowels. In figure 2.6, the chorus compensated well for the darker vowels by adding more brightness. There is consequently no drop between the first [i] and the three ensuing [E] vowels (from 0 to 6.5s.). However, when the chorus returns to the [i] of leison (7 to 8.5s.), they sing it sharp, as they are still in an acoustical attitude of compensating for the intrinsically lower pitch of the darker vowels. On the other hand, it is readily noticeable that this same rise was present in the first example (fig.2.5). Just as adding more chiaro (brightness) to the darker vowels has shown to be helpful in stabilizing their fundamental frequencies, it might be assumed that adding more oscuro (darkness) to the brighter vowels would offset their tendency to be sharp. As the chiaro aspect of this balanced resonance concept was highlighted in these experiments, this hypothesis remains to be tested more precisely in the future. However, in the majority of cases, chiaroscuro resonance balancing seems to provide choral singers with an effective and accessible tool to counteract the natural tendency to rise and fall in pitch according to whatever vowel they are singing.

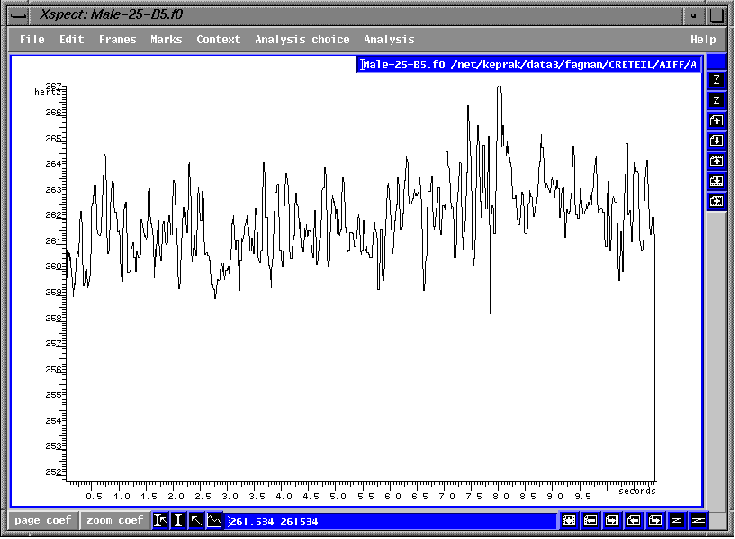
fig.2.6 Kyrie e leison after (same choir as in fig. 2.5 - see comments above).

Another important element of chiaroscuro resonance balancing is the effect that it can have on the vocal timbre and amplitude of an ensemble. By employing a full and balanced "bright-warm" sound in which all of the vowels are fully resonant, the vocal tract places itself in a position to resonate in harmony with the sung pitch, thereby amplifying partials which should be part of the tone while filtering out those which should not. The following sound example illustrates how choristers can employ this principle to exploit more of their resonance potential, thereby improving their timbre and increasing their amplitude, without there being a pejorative effect on vocal ensemble.


*** SOUND EXAMPLE (before) ***
*** SOUND EXAMPLE (after) ***

If we extract the very beginning and ending of the preceding examples and link these together, it becomes evident that employing chiaroscuro resonance balancing has helped the ensemble retain not only a homogeneity of timbre and amplitude through to the end of the phrase, but also of consistent pitch.

*** SOUND EXAMPLE (before) ***
*** SOUND EXAMPLE (after) ***

It is important to note that the ensemble was given the same pitch immediately prior to each example, G3 at 196Hz, the frequency of the 'after' segment.

III. La nota mentale

In the two examples which follow (fig. 3.1 and 3.2), we can see the spectral effect, and hear the difference in sound, when the principle of la nota mentale is put into practice. According to this tenet, singers are to imagine and prepare all of the vibratory and resonance energy required for the onset of phonation upon inhalation, before a sound is ever made. The preparation of the proper environment in the torso which leads to effective breath management, or appoggio, is also to be incorporated into each inhalation. This principle was one of the pillars of bel canto vocal pedagogy.
The sound files which led to the Fourier Transform analyses in figure 3.1 are extracted from the very beginning of a phrase of the well-known American song "Shenandoah" ("Away you rolling river"). Although the amplitude of the fundamental frequency is the same in both versions, it is evident that there is very little upper harmonic activity in the `before' (red) version. Conversely, with the implementation of the technique of la nota mentale (black spectrum), upper formant peaks are readily apparent within this very brief beginning (first 1/2 second of the attack). It is well known that these upper formants enable the sound to carry in a large hall and add colour to the vocal timbre.

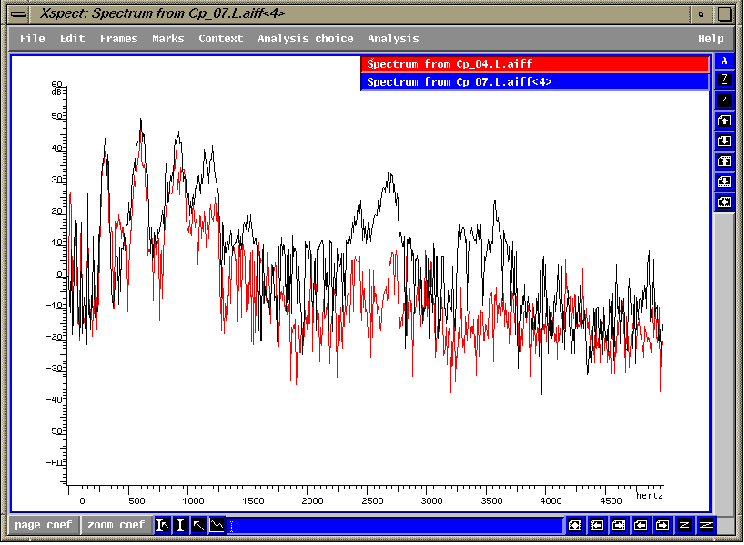
fig.3.1 Male chorus singing Eb4, first half second of attack. Red is before, black after.

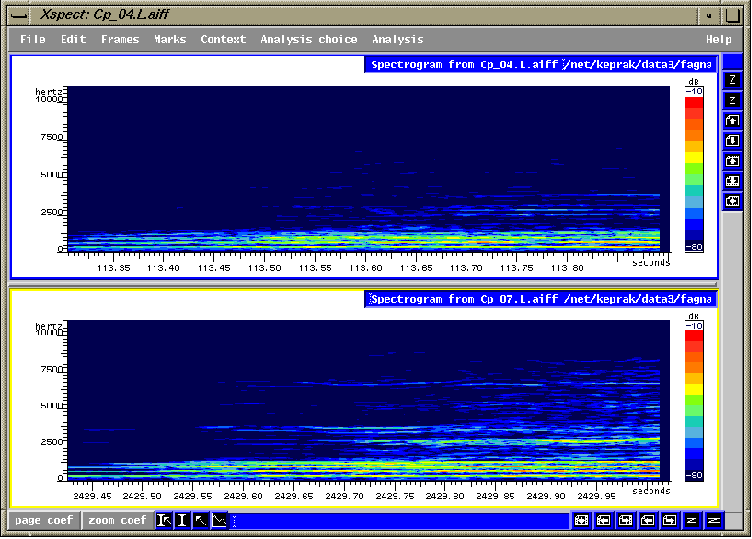
fig.3.2 Same example as figure 3.1 displayed with a spectrogram, showing the time element along the 'x' axis and frequency on the 'y'. It is easily discernible that increased upper harmonic activity is present much sooner in the second window, after the ensemble has integrated the principle of la nota mentale into its singing.

Figure 3.3 (taken from same sound excerpt as figures 3.1 and 3.2), displays the increased singer's formant energy present at the very beginning in the bel canto version (dotted line) as well as the more rapid increase in this energy until there is a 24dB difference in this frequency band separating the two versions after the first half second.

fig.3.3 A measure of the evolution of harmonic energy in the region of the singer's formant for the first 0.4 seconds of a phrase. Same sound excerpt as figures 3.1 and 3.2. Dotted line is 'after.' Once again the vertical axis should actually read decibels, not Hertz.

*** SOUND EXAMPLE (before)***
*** SOUND EXAMPLE (after)***

The breathy start and ill-prepared vowel of the 'before' version compromise both the vibratory and resonance energy potential of the choir, whereas this same acoustic energy is maximized after working aspects of la nota mentale. At the very beginning of the window (figure 3.3) there is a difference of 9dB which separates the two versions. 0.35 seconds later the gap has widened to 24dB, demonstrating that the singers' vocal preparation has paid acoustical dividends. Although the choral blend is somewhat compromised in the 'after' example, as one voice takes the principle to the extreme, the immediate improvement in timbre and strength is nonetheless an important factor of la nota mentale. Having more time, of course (all experiments were limited to a 2-hour period), the blend issue could be effectively addressed.

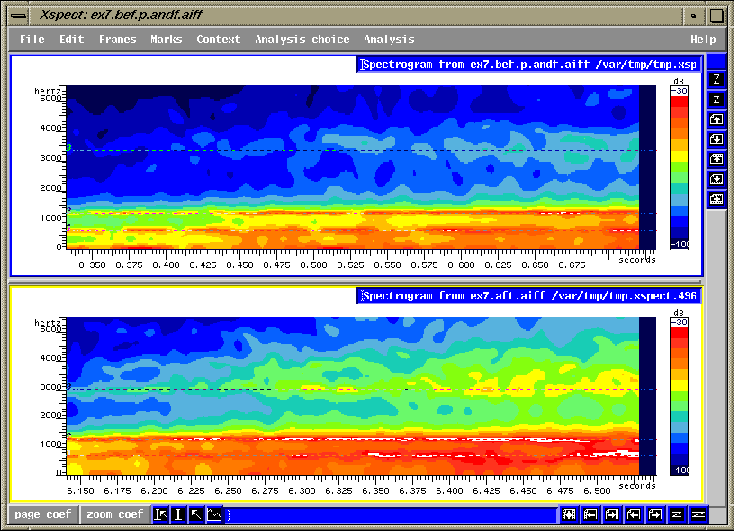
fig.3.4 University mixed choir singing the first 0.4s. of a four-part chord (alleluia).

Of note in figure 3.4 is the presence of all lower formants (indicated by dark, horizontal lines) from the outset of the sound (the graph moves temporally along the 'x' axis from left to right). The early appearance of the lower formants will assure that the vowel is immediately recognizable. The superior harmonic energy in the 3kHz region is also evident in the second version. The presence of the mid-green as opposed to mid-blue suggests an intensity increase of 15dB in this band. It is also evident that this energy enters sooner and in a more stable fashion in the 'after' version.
In order to achieve this, the ensemble was encouraged to inhale with the shape of the vowel already prepared and to have the breath drop low into the body, keeping a feeling of open emptiness (devoid of breath pressure and ready to be immediately filled with vibration and resonance) in the chest, throat and head as admonished by the great bel canto masters. Just as a light turned on in a dark room would immediately fill the entire room with its light, the choristers were encouraged to immediately fill their entire vocal tract with a well-prepared, vibrant and chiaroscuro vocal tone.
Aside from greater harmonic activity and more immediate strength to the sound, the principle of la nota mentale provides greater accuracy of intonation at the beginning and ending of chords and phrases as can be heard in the following example:

*** SOUND EXAMPLE *** (before) -mixed choir singing the end of a four-part chord on alleluia
*** SOUND EXAMPLE *** (after)

In the 'after' version of the previous sound example, the singers were asked to keep their mouths in the position of the vowel until after they were finished singing, as opposed to allowing the vowel, hence the intonation and resonance energy, to collapse as they end the sound as they do in the first version. They were also encouraged to maintain full vibratory energy to the very end of the chord which was to be immediately followed by an inhalation rather than an exhalation. This process ensures that the end of the vibration is not dispersed by outward breath flow caused by a relaxation of the vibration-breath relationship. One can hear how all of these elements allow the room to add a seemingly longer and different type of reverberation to the superior energy of the second ending.

IV. Messa di voce

Just as the principle of la nota mentale assures a consistency of vocal energy and accuracy of intonation at the beginning and end of phrases, that of messa di voce is designed to maintain this same consistency within the context of changing dynamics. Of special interest to the early bel canto masters was the art of performing the perfect diminuendo, one in which the voice effortlessly diminishes in volume to the softest point while still maintaining all of its colour and carrying power. It has often been remarked, in both the concert setting as well as in scholarly journals, that vocal ensembles often relinquish vocal intensity and stability as they diminish in volume.
The principle of messa di voce upholds that one must maintain ("spin", "ride") exactly the same vibration along the full course of the diminuendo without allowing any breath flow to compromise this vibration. This is easier to accomplish if one thinks of inalare la voce, that is if one can maintain as much as possible the feeling of 'inhalation' in the body as opposed to 'exhalation.' This attempt seeks to delay the collapsing action of the intercostal muscles which help to expel carbon dioxide from our lungs. With the breath out of the way and the singers concentrating on maintaining consistent and collected vocal vibration (which will aid in stabilizing the fundamental frequency), they have only to add to this the sustaining of complete chiaroscuro resonance to effect a successful diminuendo.

The EnerBande program is very helpful in detecting the maintenance or loss of upper harmonic energy with changes in amplitude. Figure 4.1 represents two decrescendos, the grey being before the ensemble worked on messa di voce, the heavy black line, after. It is clear that as time progresses and volume diminishes, the gap in the volume/energy ratio widens and the bel canto version displays its acoustic advantages.

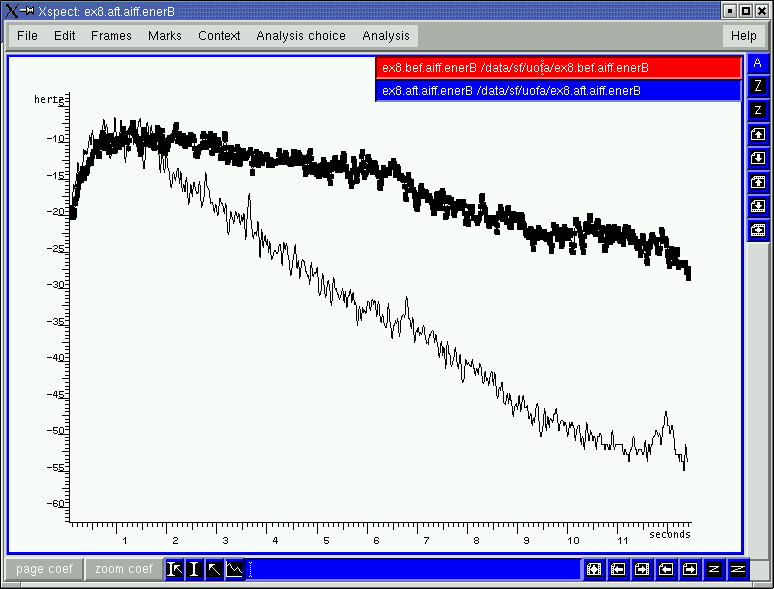
fig.4.1 A decrescendo sung by a mixed choir. Grey=before, Black-dotted=after. 'Y' axis should read decibels, not Hertz.

*** SOUND EXAMPLE (before) *** Choir singing long decrescendo on [a] vowel.
*** SOUND EXAMPLE (after) ***

The following analyses represent short, vertical portions of figure 4.1 that have been extracted and displayed separately. Each of these windows roughly corresponds to the dynamic designations of forte, mezzo forte, mezzo piano and pianissimo respectively and helps to more accurately measure the ever-widening margin between the two versions. It is the distance between the two lines (red-dotted line is the bel canto version), and not their undulations, which is important.

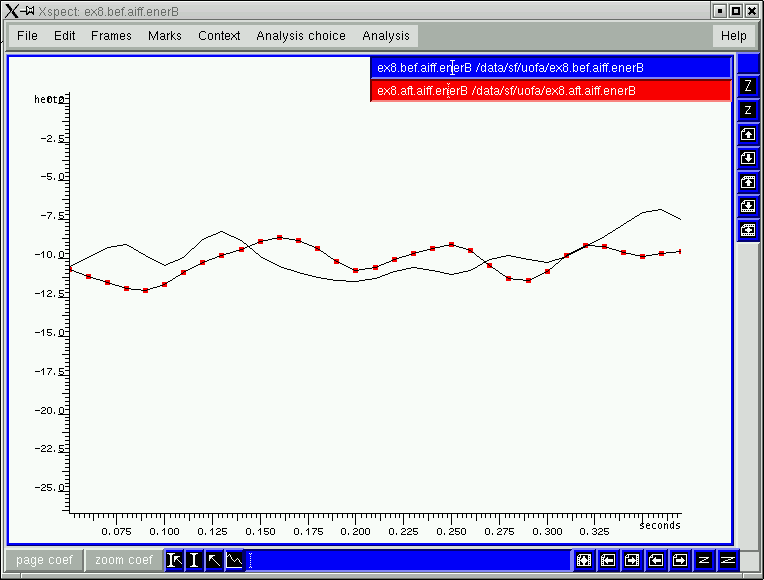
fig.4.2 Forte portion of the diminuendo.

This ensemble was able to produce a forte sound with plenty of energy prior to having worked on the bel canto principles. There is consequently no quantifiable difference in upper harmonic energy between the before and after versions of this portion of the exercise. In listening to the sound example below, however, one can notice a fuller sound and improved vowel quality in the 'after' version.

*** SOUND EXAMPLE (forte portion, before)***
*** SOUND EXAMPLE (forte portion, after)***

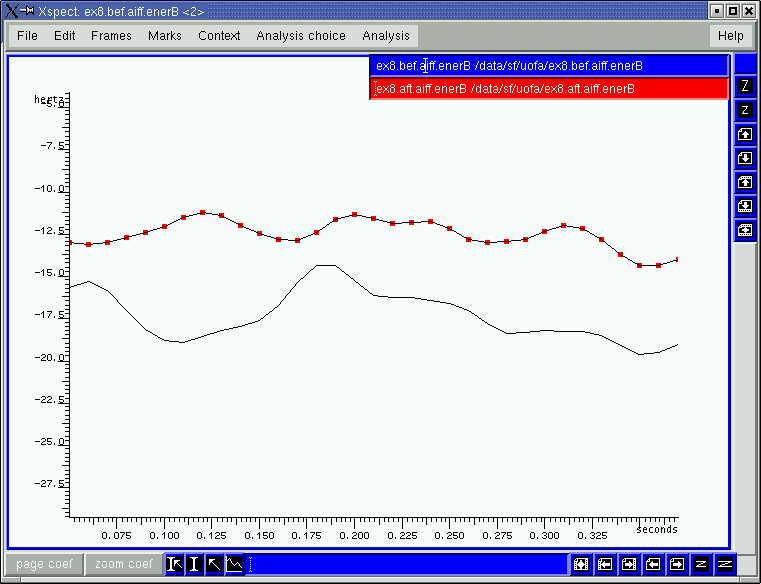
fig.4.3 Mezzo forte

Mezzo forte: After having worked the principle of messa di voce, the ensemble retains 4.35 decibels more upper harmonic energy than before (figure 4.3).

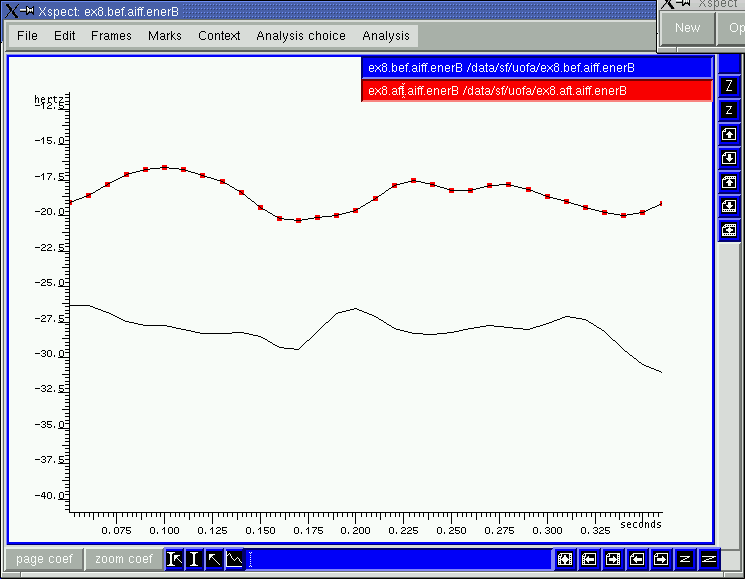
fig.4.4Mezzo piano

The gap widens to 9 decibels for mp.

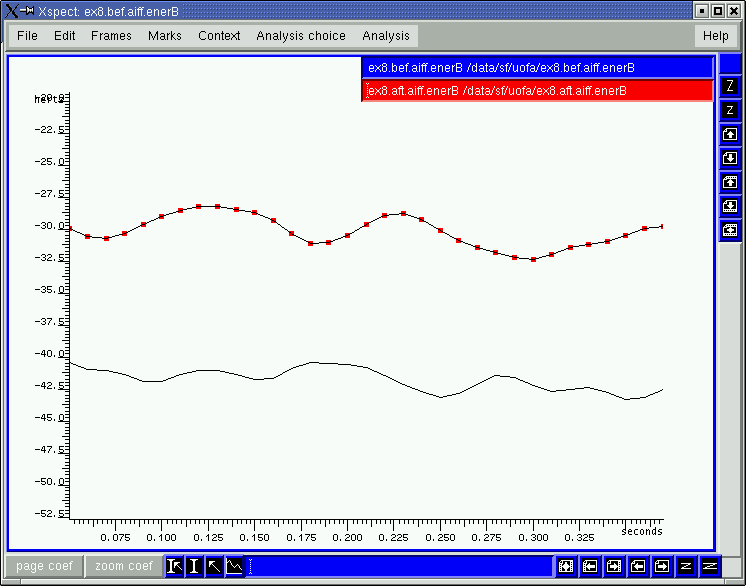
fig.4.5 Pianissimo

A difference of 11.5 decibels has developped by the time the choir reaches the dynamic marking of pianissimo.

*** SOUND EXAMPLE (pianissimo portion, before)***
*** SOUND EXAMPLE (pianissimo portion, after)***

The choir has clearly been able to maintain greater energy of vibration and a more complete timbre in the second sound example. The 11.5 -decibel disparity in the pianissimo version is also quite revealing considering that the forte versions began at the same intensity level in this band.

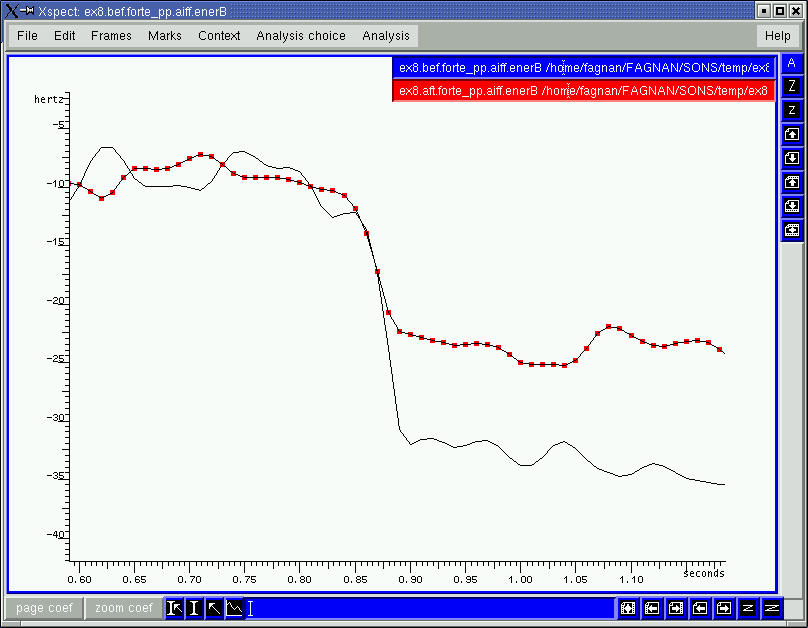
fig.4.6 Comparison of upper harmonic energy during abrupt shift from forte to pianissimo (at 0.9s). Red-dotted line=after bel canto work.


In the figure above (4.6) and corresponding sound examples below, the middle portion of the preceding diminuendo has been removed. The resulting abrupt juxtaposition of forte and pianissimo illustrates the difference in quality of sound as well as the disparity in upper harmonic energy between the two examples. Evident in figure 4.6 is the greater amount of singer's formant energy retained after the principle of messa di voce has been worked. One can also hear that the ensemble has dropped in pitch in the first example as much of its vibratory energy has been dispersed by superfluous breath flow.

***SOUND EXAMPLE (before)*** (mixed choir, abrupt shift from forte to pp portions of the same diminuendo)
***SOUND EXAMPLE (after)***

It is apparent in the examples of this section that the bel canto principle of messa di voce can be very useful in helping vocal ensembles retain a greater amount of acoustic energy the softer they sing, once again providing them a tool leading to greater vocal and acoustical efficiency in choral singing.

CONCLUSION:

Although the application of bel canto vocal principles can lead to occasional problems with blend, it is believed that when applied with discretion and worked into a choir's sound, these standards can be very helpful in advancing a choir's vocal technique without compromising choral ideals. The acoustic benefits provided by the implementation of these principles within the choral context would seem to far outweigh the disadvantages. It is clear that encouraging an ensemble to improve its ability to sing with consistent and collected vocal vibration will do much to improve the group's sound and harmonic spectrum. It has also been shown that using consistent chiaroscuro resonance can have a very positive effect on group intonation while improving overall timbre. The application of messa di voce in softer dynamics has also been established as a useful tool in helping ensembles to sing with greater efficiency and a more consistent sound in softer dynamics. It is hoped that more choral conductors will look to these principles in the future when developping the vocal sound and technique of their ensembles.

*** SOUND EXAMPLE (before)***
*** SOUND EXAMPLE (after)***

Notes :

1. Sten Ternstrom et al. "Articulatory F0 Perturbations and Auditory Feedback." Journal of Speech and Hearing Research , 31 (June, 1988), 190.
Sten Ternstrom. "Physical and Acoustic Factors that Interact with the Singer to Produce Choral Sound." Journal of Voice , 5,2 (1991)137,8.
Johan Sundberg. The Science of the Singing Voice. (Dekalb, Il., Northern Illinois University Press, 1987), 143.