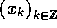 we rebuild the original analog signal
we rebuild the original analog signal .
.
The ideal FDDF may be defined by reference to analog time-delay
filters as described in diagram 1
[3]. From a time sequence 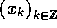 we rebuild the original analog signal
we rebuild the original analog signal![]() , then we delay it and finally we
re-sample it in order to get
, then we delay it and finally we
re-sample it in order to get  .
.
This definition is no longer consistent if there is
some residual energy at the Nyquist frequency. For instance the
sequence 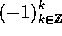 may be the sampled
version of any of the
may be the sampled
version of any of the 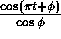 functions. Furthermore a shifted cardinal sine isn't absolutely
summable which means that FDDFs aren't BIBO
functions. Furthermore a shifted cardinal sine isn't absolutely
summable which means that FDDFs aren't BIBO![]() . For these reasons, we
shall limit the study of FDDFs only for input sequences
with no component at the Nyquist frequency. In this
subspace, FDDFs are consistent and BIBO.
. For these reasons, we
shall limit the study of FDDFs only for input sequences
with no component at the Nyquist frequency. In this
subspace, FDDFs are consistent and BIBO.